Notes on your Favorite Mathematician’s Favorite Mathematician

Recently I got to attend a talk from Sir Andrew Wiles. Whoa.

The talk was on Dirichlet. So, now you know your favorite mathematician’s favorite mathematician.

This post is supposed to be a collection of the notes I wrote down during the talk.

So, Dirichlet has been Wile’s favorite mathematician for the majority of his career. Dirichlet’s full name was Johann Peter Gustav Lejenne Dirichlet. He went to study in Paris when he was about 17 years old, and then published his first paper when he was 20. It was on Fermat’s equation, specifically finding solutions to \(x^k + y^k = z^k\) when the solutions lie in the nonzero integers! He managed to solve it for the 5 case, and then later did it for 14.

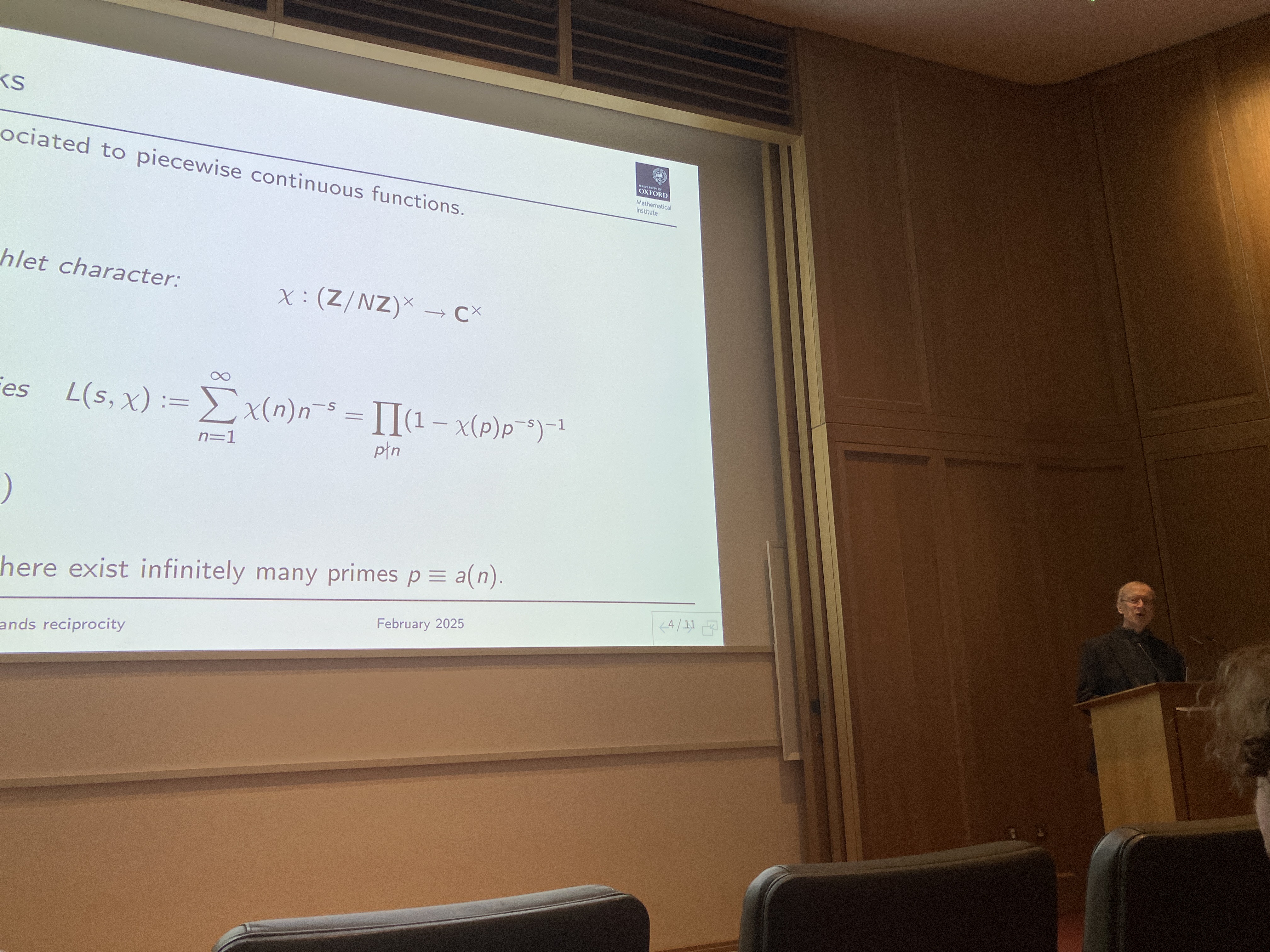
This, of course, brought him fame, but it also brought him closer to other mathematicians, such as Fourier. When he was 24, he ended up publishing a paper on the convergence of fourier series over piecewise continuous functions.

And then when he was 32 he did his big arithmatics progression theorem:

Let a, n be positive and coprime. Then a, a +n, a + 2n, a + 3n, … has infinitely many primes.

To do this, he had to first venture into using characters (and now we call them Dirichlet Characters) and L-series, which are built on these characters. This makes Dirichlet the father of analytical number theory.

…But then he had to go do his final end of his degree by giving a speech and answering questions… all in Latin! That’s a bit silly.

Back to personal details. He ended up marrying into a very musical family, though I don’t remember which one. However, this family had many connections to other mathematicians, such as Kummer and two others mentioned by Wiles.

Then it was around this time that Kummer did his false proof of Fermat’s Last Theorem. (I just want to point out that Wiles mentioned this. It feels kind of fun.)

Dirichlet was the one who pointed out that this proof didn’t work. I remember seeing this false proof back in Ohio during Ross Math Program in 2023, during a class field theory lecture taught by Bartu Bingol. If you want to learn the details on this false proof, more can be found here. The main reason it doesn’t work is because Kummer assumed that he was operating over unique factorization domains, except there are many such rings that aren’t!

Now, we segway.

Wile’s then goes to talk about Pell’s equation, which is of the form \(x^2 - Dy^2 = 1.\) To solve this, most people used the convergents of continued fractions to find the fundamental solution and then generated the rest from there. I had seen this before in Continued Fractions at Proof School.

But now this is the part of the notes where I’m not actually sure what I wrote and why I wrote it.

Dirichlet has another theorem, called the Approximation Theorem, on which my Ross family and I gave a talk on. This is what allows you to almost immediately show the existence of solutions to Pell’s Equation.

A binary quadatic form is some function \(f(x, y) = ax^2 + bxy + cy^2.\) We let \(\disc_f = b^2 - 2ac.\) We have an equivalence relation on quadratic forms by considering the right group action of multiplication from SL_2(Z). If you want to see more on this, I strongly reccomend reading this.

The class field number is then the number of reduced binary quadratic forms of a given discriminant D. This is in general important, and what Dirichlet did was find a closed formula for class numbers in certain cases! One of his formulae uses the Legendre Symbol (QR says hello), and the other formula is utilized when your discriminant is some prime equal to 1 mod 4. It had sine in it. IT HAD SINE IN IT???

As you can tell, I’ve reached a point of I’m not actually totally sure what’s going on here. So, now I’m just going to recount from my notes.

This motivated looking at the zero of the L-series at 1, which to write it out uses zeta functions.

Wiles said Dirichlet developed pigeonhole principle? Which is. Whoa. Yeah, your favorite mathematician’s favorite mathematician. Funnily enough he called it Schubfachprinzip, though I won’t be using that in my proofs.

Both Riemann and Dedekind of analytical fame were students of Dirichlet. Whoa!

Now for some more recent mathematics. Consider some curve C defined by the equation p(x, y) = 0. A theorem from 1983 shows us that it has finitely many solutions over the rationals if the genus of C is two or more.

So, this has motivated the study of elliptic curves, especially the Birch and Swinerton-Dyer Conjecture (a Millenium Problem). What Mordell-Weil tells us is the rank of an elliptic curve, which is the size of the basis of the group of rational points on the curve, is finite. So, that’s really, really nice, but we want a general method for finding the rank.

The conjecture tells us that the rank of the curve is the multiplicity of the zero of the curve’s associated L-function at 1. This generalized L-function over an elliptic curve was studied by Gross-Zeiger and Kolyvagin.

What we have now is if this function is nonzero at 1, then there are finitely many solutions.

If this function is 0 and has nonzero derivative at 1, then it has infinitely many solutions.

The conjecture is that this generalizes, if that the first couple of derivatives are zero and then eventually nonzero, then there are infinitely many solutions. This would require the B&SD Conjecture.

Now, we hit the end. There’s this quote he said:

So I was giving this lecture, say about, 30 years ago. I was talking to Atiyah after, and he said “How do you feel that number theory is just going to become a part of physics?” Some physicist then replied “no, I think physics is going to become a part of number theory.”

I believe this happened because some physicist in the crowd asked how he felt about Quantum Field Theory being used in the Langland’s program. He laughed at first and said “whatever works!”

He had Langland Reciprocity at the bottom of the slides, so I asked, and he explained it as QR is the bones of number theory, and Langlands Reciprocity is one major goal of the Langland’s program that wants to show reciprocity laws hold elsewhere. He stated that it states that as L-series come from modular forms, that means that they should always have reciprocity laws.

When asked about modern mathematics, he said that he felt Scholze was doing interesting work in algebraic geometry, modern number theory seems to be relying on ergodicity, and he feels as though modern day p-adics are similar in impactfulness as complex numbers back in the beginning of the 19th century.

When asked about what books he liked reading, he said David Copperfield.

When talking about math research, he said he wanted to work on FLT as a PhD student. His advisor warned him against this and so he spent it working on a different problem:

Say we have n points in R^3. Each two points generate a line, so say there are l lines, and all 3 points generate a plane, so say there are p planes. Then we have the bound l^2 < np. (I believe there was a constant of 2 somewhere in here, but I’ve forgotten.)

He mentioned after that it was solved now.

He said that forgetting is an important part of mathematics, and that the new age of mathematics with computers will be interesting.

The forgetting thing has stuck with me. I feel like I don’t remember most of the math I did in high school and that deeply troubles me, so I spend time going back and revising why I hold truths in my head until I can hold them in my heart.

I worry that I didn’t actively capture all the math/facts as correct as possible; if there’s a complaint, please email me!

My biggest takeaway from this is that deeply engaging with Ross was very nice, and something I should’ve done more of. If you are interested in this mathematics, I would highly reccomend applying! It was a major reason of why I was able to stay afloat during this talk.